Smoothed Quadratic Energies on Meshes
Martinez Esturo, J. and Rössl, C. and Theisel, H.
Abstract
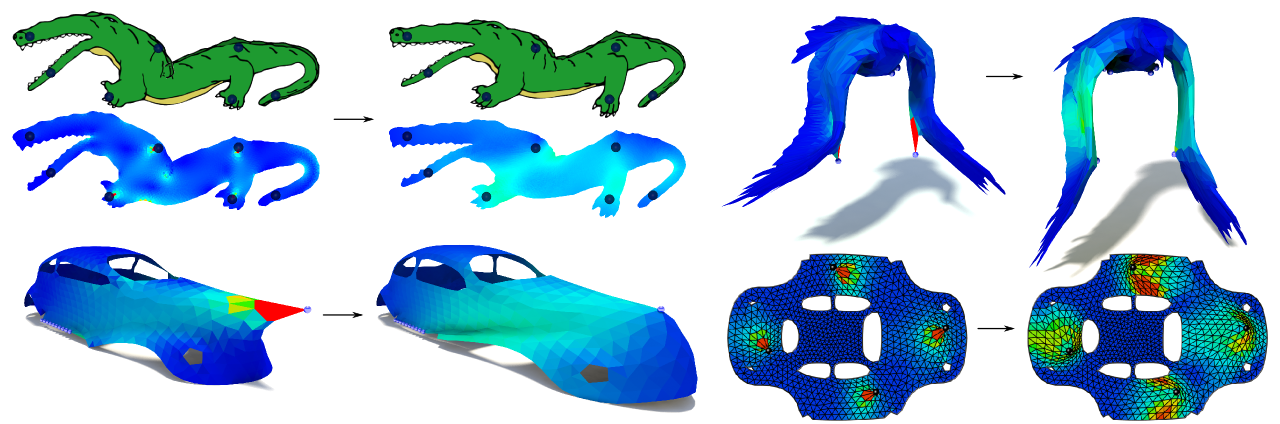
In this paper, we study the regularization of quadratic energies that are integrated over discrete domains. This is a fairly general setting, which is often found in but not limited to geometry processing. The standard Tikhonov regularization is widely used such that, e.g., a low-pass filter enforces smoothness of the solution. This approach, however, is independent of the energy and the concrete problem, which leads to artifacts in various applications. Instead, we propose a regularization that enforces a low variation of the energy and is problem-specific by construction. Essentially, this approach corresponds to minimization w.r.t. a different norm. Our construction is generic and can be plugged into any quadratic energy minimization, is simple to implement, and has no significant runtime overhead. We demonstrate this for a number of typical problems and discuss the expected benefits.
Video
BibTeX
@article{MartinezEsturo2014,
title = {Smoothed Quadratic Energies on Meshes},
author = { {Martinez~Esturo}, J. and R{\"o}ssl, C. and Theisel, H.},
journal = {ACM Trans. Graph.},
number = {1},
pages = {2:1--2:12},
volume = {34},
year = {2014}
}